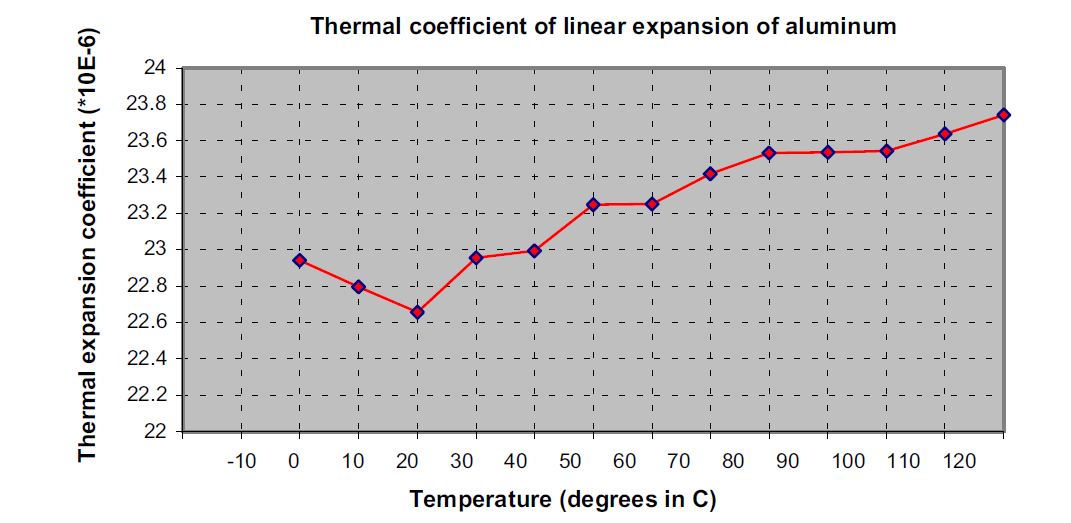
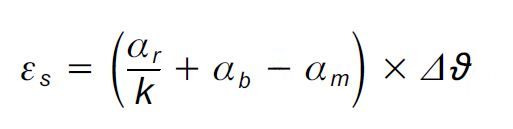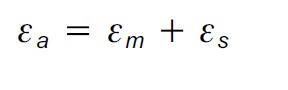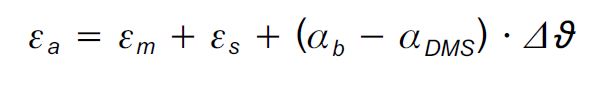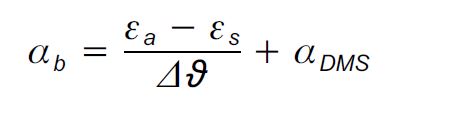If you calculate αm for the specified interval, you obtain 23.19 *10-6/K, which corresponds to a deviation from the theoretical value of 0.19 *10-6/K (0.84%).
To run the experiment, it is first necessary to install several strain gauges on the object under investigation (to attain experimental reliability). The sample must be flat in the direction of the measuring grid.
In the next step, the strains are determined subject to the temperature. Care must be taken to ensure that thermal equilibrium is established.
First εa-εs is calculated. To determine the thermal coefficient of linear expansion, you subtract the two calculated values (εa-εs ) from each other and divide this by the corresponding temperature interval. The coefficient of thermal expansion αDMS as per the pack data must then be added to this.
Example: In the interval from 20 to 40 degrees, the coefficient of thermal expansion is calculated as follows (using calculation shown in Formula 4):