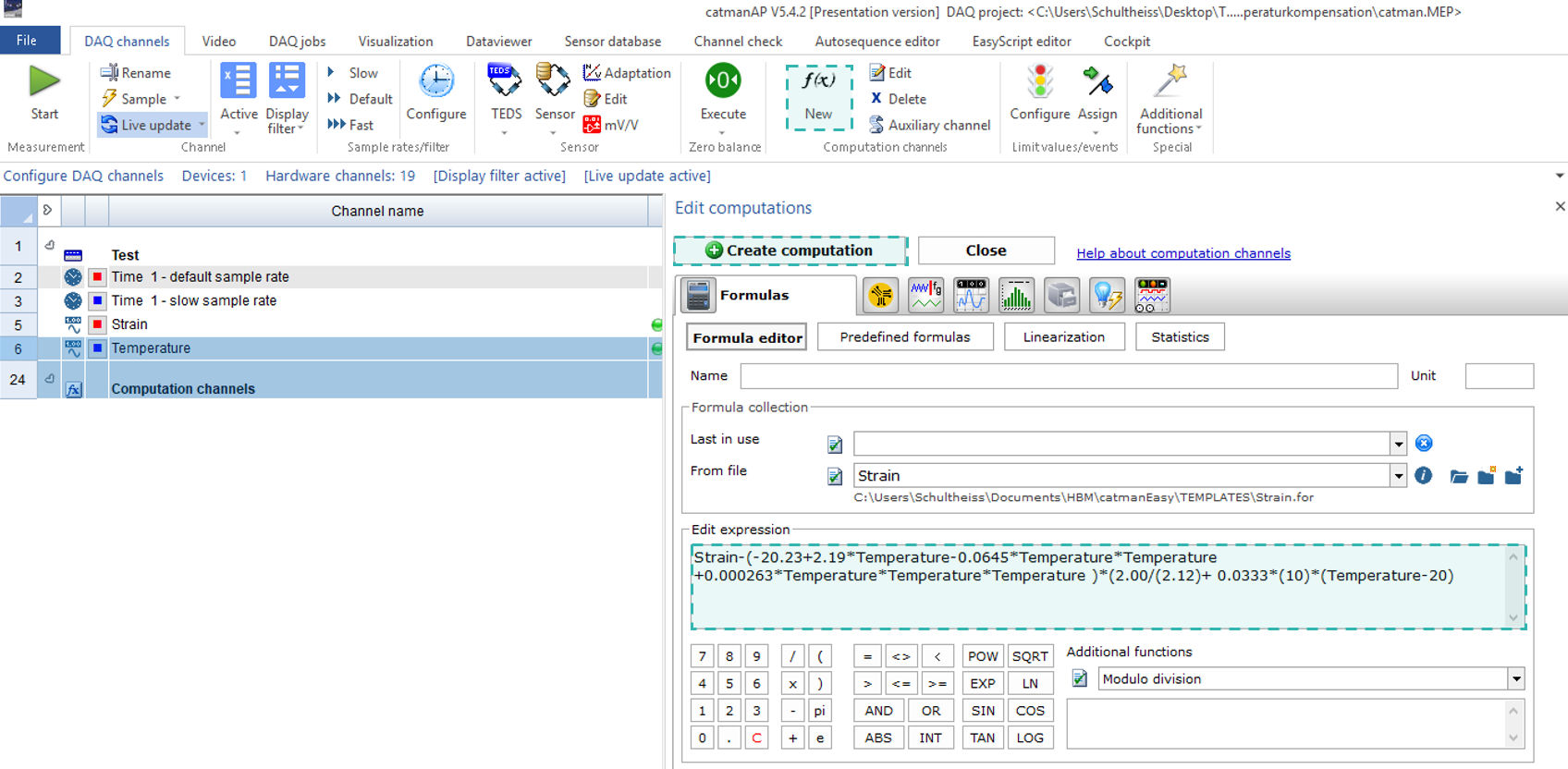
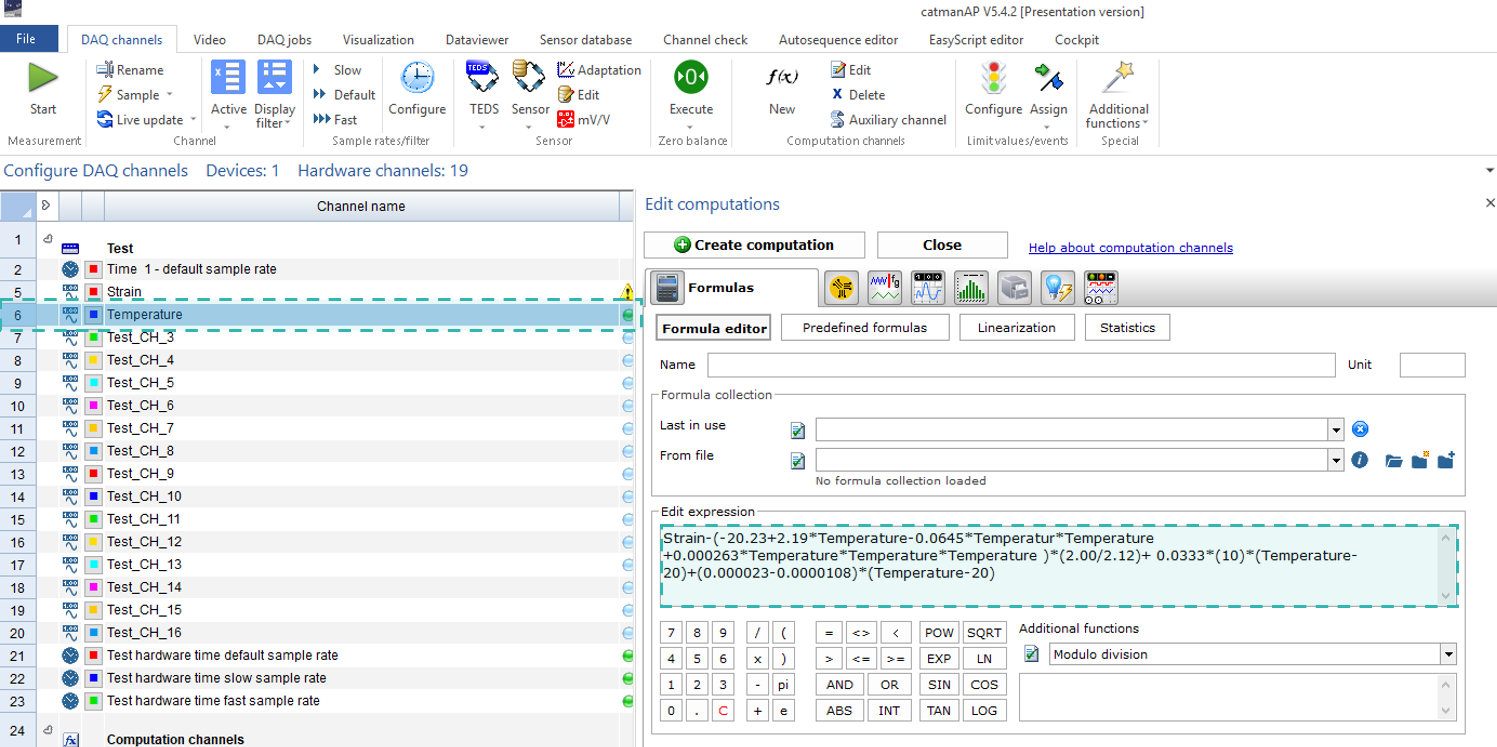
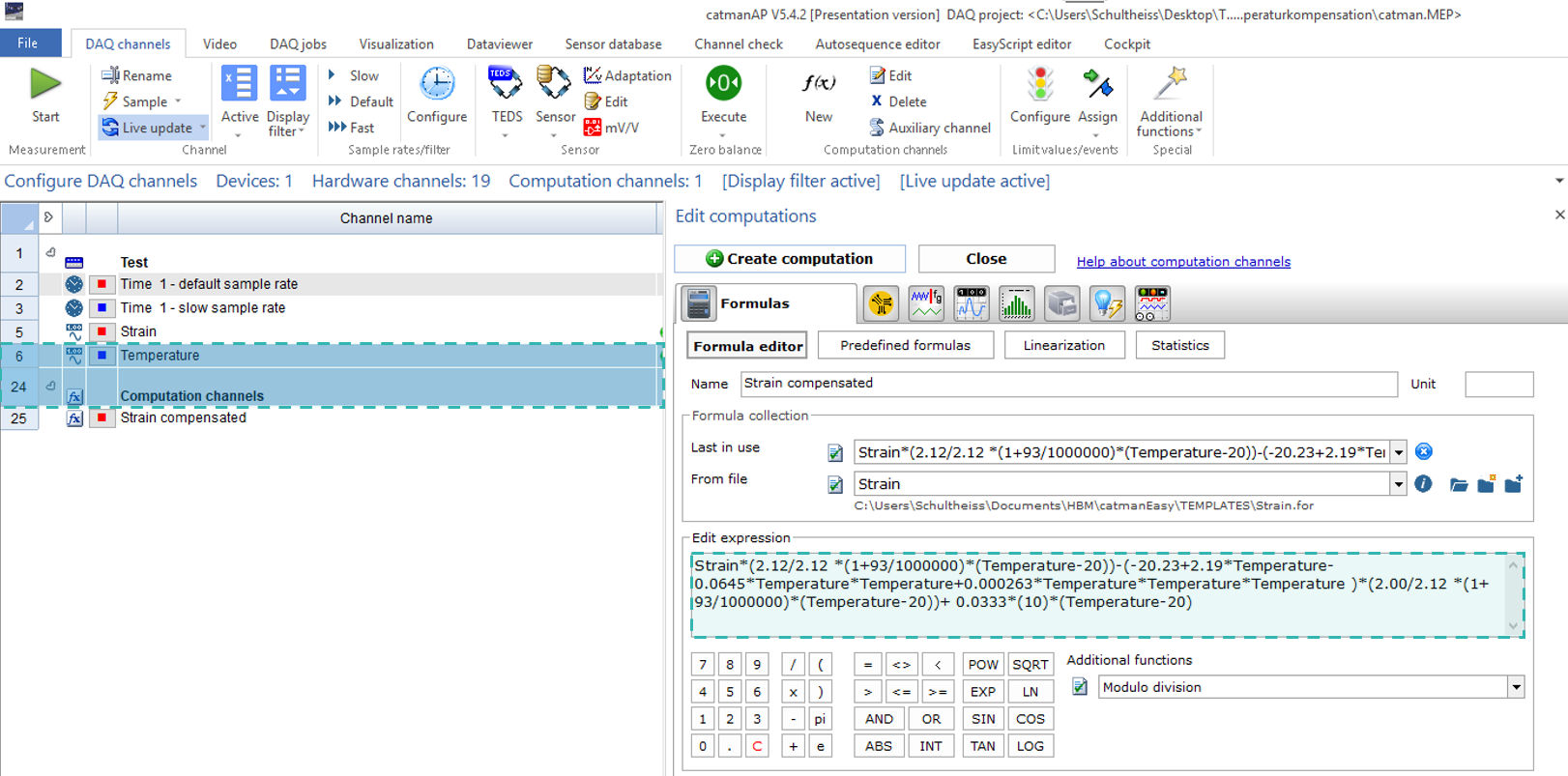
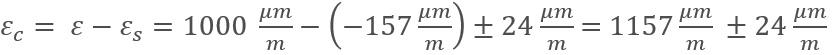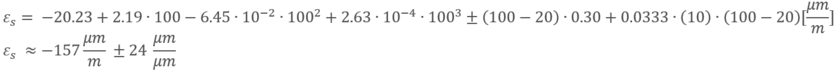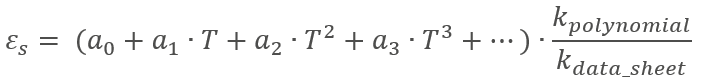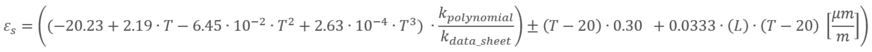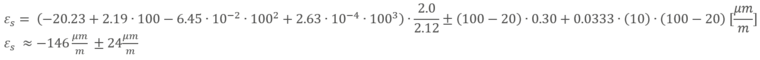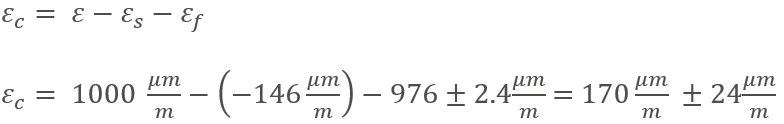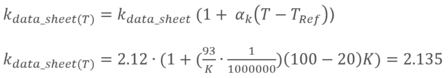Temperature Compensation of Strain Gauge Quarter Bridges Temperature Compensation of Strain Gauge Quarter Bridge | HBM

Clear and Understandable: Temperature Compensation of Strain Gauge Quarter Bridge Applications

To ensure accurate measurement results even under changing ambient conditions, temperature compensation of quarter bridge strain gauge applications need to be established. Several different temperature factors influence the physical strain on its way to digitalization, such as:

  • The mutual influence of thermal material expansion differences on substrate/base material and strain gauge grid material
  • The ohmic resistance of the lead wires
  • The temperature dependency of the gauge factor by self-heating of the strain gauge
  • The temperature dependency of the Youngs modulus

 

These temperature influences can be corrected by using software packages designed for strain gauge applications such as catman Easy/AP from HBK. We will give you a clear and understandable link between theory and practice of temperature compensation for quarter bridge strain gauge applications.

Strain gauges from HBK are shipped with a data sheet, containing all relevant parameters to ensure the accuracy of the measurement. The HBK strain gauge data sheet has the following layout and displays the parameters for compensation in a chart and formula. Annotation: In this example, two curves are displayed in the data sheet. One curve represents the thermal response of the strain gauge itself while the other curve represents the thermal behavior of the gauge including 2-wire leads. 

HBK Strain Gauge Data Sheet
HBK Strain Gauge Data Sheet (click to enlarge)

The temperature response of a strain gauge depends on:

  • The temperature expansion coefficients of the substrate/material
  • The temperature expansion coefficient (CTE) of the strain gauge grid
  • The temperature coefficient of the ohmic resistance of the strain gauge grid
  • The temperature coefficient of the gauge factor k

 

In practice, the thermal output strain is simply measured and extracted in a temperature chamber under accurate conditions. The result of this measurement reflects:

  • The compensation of temperature expansion of the substrate/material by the strain gauge properties
  • A residual error that cannot be compensated but corrected for highly accurate measurement demands

The residual error can be determined from the measurement and represented by a polynomial εs, which ideally would always give zero as the result, regardless of temperature. But in practice there is a range around the reference temperature, where it is optimised close to zero during production of the strain gauge.

Application Example

We will perform an example calculation to demonstrate how thermal compensation in quarter bridges can be established considering the most important influences. We take the following setup:

  • Transducer: Strain gauge of type 1-LY11-10/120 installed on a material in a test chamber with the test condition of 100°C. To connect the transducer with the amplifier, 10mm leads, a solder terminal and a 4-wire cable are used.
  • Amplifier: Strain is measured in a quarter bridge configuration by the DAQ module QuantumX MX1615B with DC or carrier frequency excitation value depending on the ambient electromagnetic environment.
  • Software: catman Easy/AP DAQ software, installed on a PC or running in the data recorder, is used to correct the temperature influences by online math.

1. Correction of Strain Value With Polynomial

Thermal compensation in catman
Thermal compensation in catman (click to enlarge)

The thermal polynomial is plotted on each data sheet. The general layout is as follows - please be aware, that the polynomial can vary:

Thermal Polynomial

 

In catman Easy/AP the thermal compensation for the strain gauge can easily be added by clicking on “Adaption” in the ribbon bar of the DAQ channels. All parameters can be found in the data sheet.

In some cases, the polynomial includes further contributors that influence the strain signal if the temperature changes:

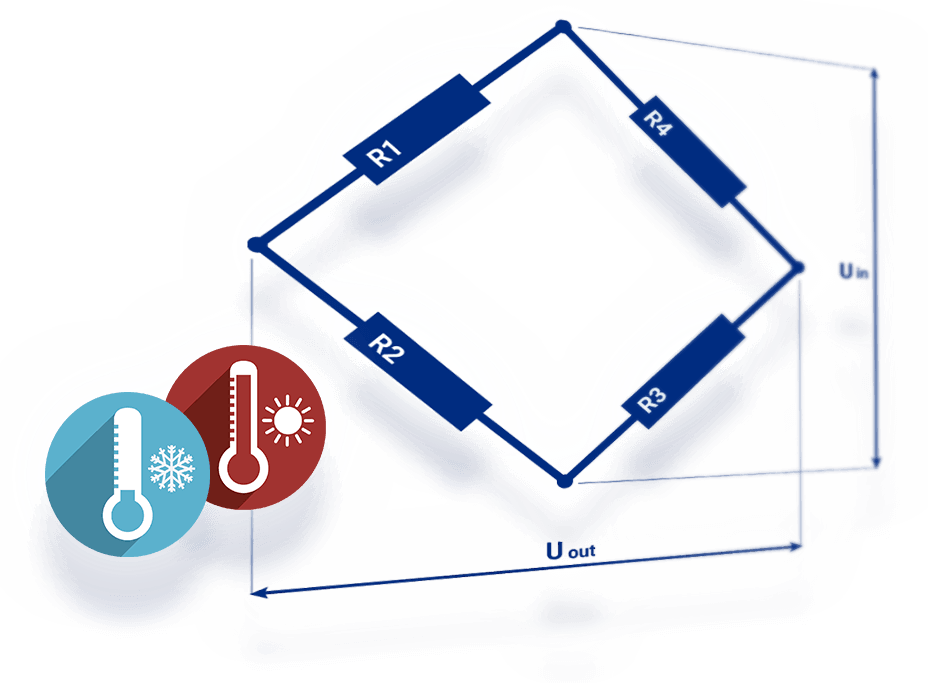
  • Influence of leads (εl): In this specific example the measurement uncertainty and the influence of 2-wire strain gauge leads connected to the measurement grid is added as well. Generally, the influence of leads must be considered as well, but it might differ between gauge types and manufacturers. If you are using our HBM-patented 3- or 4-wire technology this compensates all cable resistances, but in some cases, there is a residual 2-wire part which cannot be compensated automatically.
  • Measurement Uncertainty (εu): The measurement uncertainty is a general part which should be considered in the total calculation. Even the polynomial has some scatter which leads to this uncertainty part.

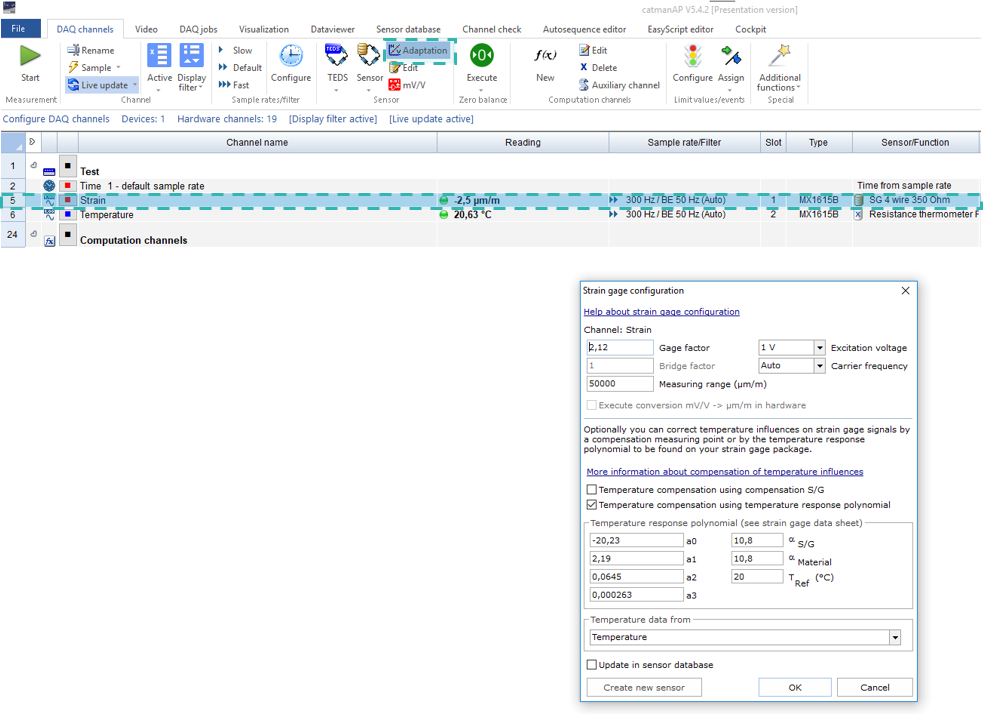
The adapted polynomial is as follows:

Thermal polyinomial including inclunce of leads and measurement uncertainty

 

Focusing on our application example it looks like:

Thermal polyinomial including inclunce of leads and measurement uncertainty

 

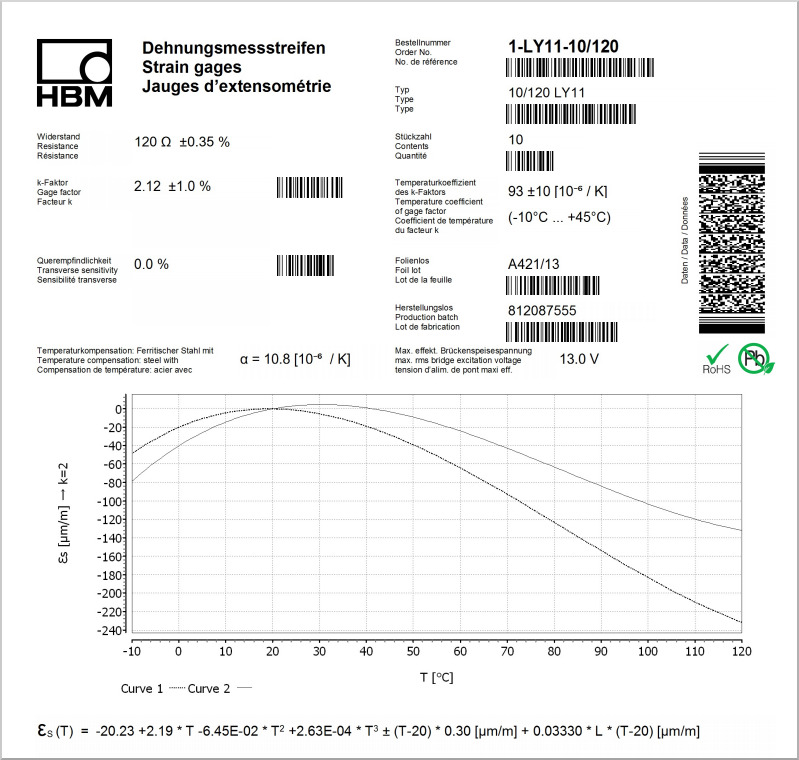
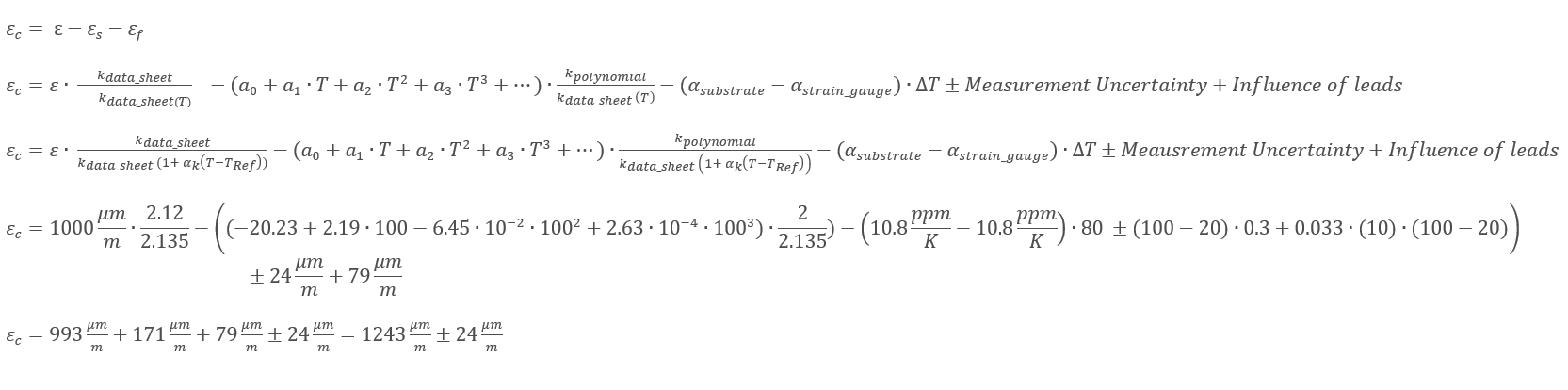
Let’s assume that the temperature during the strain measurement is constantly 100 °C (T = 100 °C) and the length of leads is 10 mm (L = 10 mm). Please be aware that the length of leads can vary. Looking at the polynomial, it demonstrates that the thermal strain has a significant impact on the result, since it is higher than 100 μm/m!

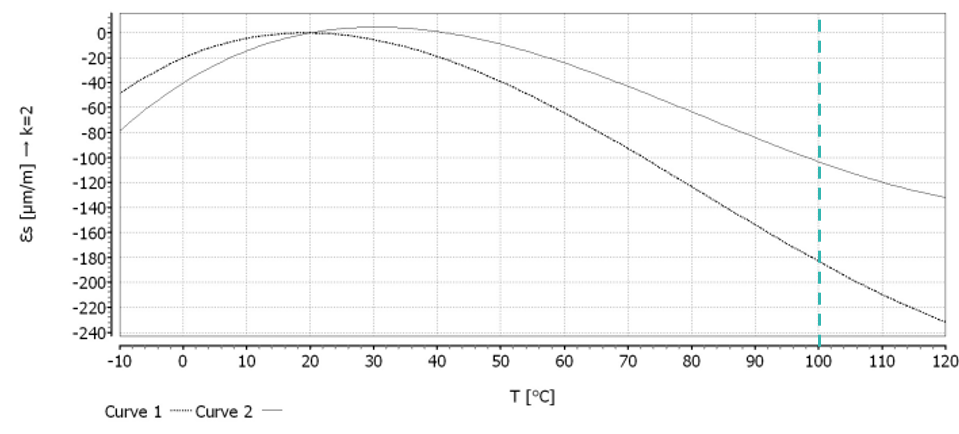
Thermal polynomial

To calculate the thermal output strain, deploy the temperature and the length of leads into the polynomial:

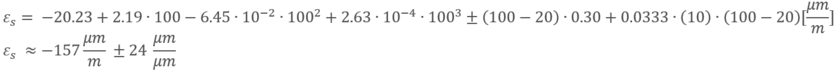
Calculation thermal output strain

 

The result fits quite well to the polynomial displayed on the data sheet. By considering the polynomial the most significant influences are taken into account, whereas only very long 2-wire cables can impact the result additionally. Since we use the 3- and 4-wire technology that compensates the impact of cable resistance, this part of the calculation is not relevant!

Superimposing the thermal strain on the measured strain now gives the corrected strain value, which only takes the mechanical strain into account:

Corrected Strain Value without thermal strain

回顶部

2. Gauge Factor Adjustment

The polynomials of most manufacturers are measured with a fixed gauge factor (or k-factor) of k = 2, whereas the real measured k-factor typically differs. This effect is mainly visible in extreme conditions, such as high and low temperatures or high strains. To compensate the variations which can influence the temperature compensation, the gauge factor printed on the data sheet should be considered.

Therefore, multiplicate the quotient of the gauge factors printed on the data sheet to correct the thermal output that can be expected with the strain gauge. In this case we have the following gauge factors:

Thermal polynomial with gauge factor adjustment

 

Of course, the thermal strain correction is only applied to the polynomial and must not be applied to additionally contributing factors as measurement uncertainty or the influence of leads:

Thermal strain correction by gauge factor adjustment

 

In this case, we have two gauge factors (kpolynomial = 2.0 and kdata_sheet = 2.12). The thermal strain correction would be:

Thermal strain correction by gauge factor adjustment

The corrected strain signal would change to:

Corrected Strain Signal by Gauge Factor Adjustment

 

To establish this correction in catman DAQ software a new calculation channel needs to be created which consider all factors. A computation channel can be added by clicking on "New Computation Channels" and "Create a new Channel". The strain signal can be corrected by inserting the thermal strain correction polynomial into the "Edit Expression" of the new computation channel. 

Gauge Factor Adjustment with catman
Gauge Factor Adjustment with catman (click to enlarge)

回顶部

3. Deviating Temperature Coefficient of Substrate Material

In this theoretical test case, the temperature coefficient of the strain gauge matches the material perfectly. Nevertheless, in practice, there might be slight deviations between temperature coefficient of the substrate material and the temperature coefficient to which the strain gauge has been adapted. An approximate adjustment to correct the measured strain value is given by the following formula. In this case, we assume that a strain gauge adapted to ferritic steel (10.8 ppm/K) was used on an aluminium (23 ppm/K):
Deviating Temperature Coefficient of Substrate Material

 

The temperature difference must be calculated from the reference temperature and the temperature during test. The reference temperature is the temperature the strain gauge data sheet refers to and for which the parameters have been measured:

Deviating Temperature Coefficient of Substrate Material

 

This will lead to the following correction factor:

Deviating Temperature Coefficient of Substrate Material

If this is considered for the corrected strain value, the overall equation is obtained:

Deviating Temperature Coefficient of Substrate Material

 

To establish this correction in catman DAQ software a new calculation channel needs to be created oran already existing calculation channel needs to be adapted. In this application example, the already existing computation channel needs to be adjusted by the updated thermal strain correction polynomial. 

Deviating Temperature Coefficient of Substrate Material with catman
Deviating Temperature Coefficient of Substrate Material with catman (click to enlarge)

回顶部

4. Gauge Factor Variation By Temperature (Optional)

In chapter 2, the strain correction was performed via the polynomial and the gauge factor fitting. This is sufficient for most experimental tests. Nevertheless, the gauge factor (k-factor) additionally varies approximately linearly with temperature over a wide range. Therefore, a correction of the gauge factor can be considered in the uncorrected strain signal (not to be considered in the polynomial, since the temperature dependence of the gauge factor is already included in the polynomial). The temperature coefficient can be positive or negative, depending on the grid material (Constantan or CrNi (Modco).

To easily calculate the temperature adjusted gauge factor, the HBK data sheet displays the temperature coefficient as well as the needed gauge factor (kdata_sheet = 2.12).  Please check first, if the linear behaviour is valid even at extreme temperatures. In this case the temperature coefficient of the gauge factor is:

Gauge Factor Variation By Temperature

The correction formula of the gauge factor is as follows (T = 100 °C):
Gauge Factor Variation By Temperature

 

It is obvious that this effect is very small and might not be considered in the measurement since the effect is neglectable.

The temperature dependency of the gauge factor can be easily considered as part of the complete formula in catman DAQ software by adjusting the already existing computation channel.

Compensation of the gauge factor variation by temperature
Compensation of the gauge factor variation by temperature (click to enlarge)

回顶部

5. The Ultimate Thermal Correction Formula

Considering all effects described in this Tech Note, the formula for correcting the strain value is as follows:

The ultimate thermal correction formula
The ultimate thermal correction formula (click to enlarge)

回顶部

6. Glossary

εStrain signal without any temperature correction
εsThermal output polynomial from strain gauge data sheet 
εcStrain value including thermal correction
εfMismatch correction of temperature coefficient
αRTemperature coefficient of the gauge resistance [1/K]
αSTemperature coefficient of the substrate/structure [1/K]
αMTemperature coefficient of the strain gauge metal grid [1/K]
kGauge factor of the strain gauge
kdata_sheetGauge factor printed on the data sheet
kdata_sheet(T)Gauge factor printed on the data sheet including temperature correction
kpolynomialGauge factor which was used to determine the polynomial (typically 2.00)
αkTemperature coefficient of the gauge factor
a0a0 coefficient of polynomial
a1a1 coefficient of polynomial
a2a2 coefficient of polynomial
a3a3 coefficient of polynomial
∆TTemperature difference of temperature during strain measurement and reference temperature printed on strain gauge data sheet
Tref Reference temperature printed on the strain gauge data sheet
αsubstrate    Temperature coefficient of material on which the strain gauge is applied
αstrain_gauge Temperature coefficient of the strain gauge

 

Download Tech Note as PDF

 

Legal Disclaimer: TECH NOTEs from HBK are designed to provide a quick overview to a specific topic beside the usual documentation. TECH NOTEs are continuously improved and so change frequently. HBM assumes no liability for the completeness of the descriptions. We reserve the right to make changes to the features and/or the descriptions at any time without prior notice.